Research Article

DNS on the Evolution of Vortices in the Upper Boundary Layer in Transition

Yonghua Yan¹, Caixia Chen²* and Fan Yang³

¹Jackson State University, USA

²Tougaloo College, USA

³University of Shanghai for Science and Technology, China

Submission: June 24, 2018; Published: July 18, 2018

*Corresponding author: Caixia Chen, Tougaloo College, Tougaloo, Mississippi, 39174, USA, Tel: 8179087642; Email: caixchen@gmail.com

How to cite this article: Yonghua Y, Caixia C, Fan Yang. DNS on the Evolution of Vortices in the Upper Boundary Layer in Transition. Eng Technol Open Acc. 2018; 2(2): 555582. 10.19080/ETOAJ.2018.02.555582

Abstract

The ring-like vortices (or hairpin vortices) are universal vortical structures in late boundary layer transition. They play a very important role in the boundary layer transition process. In this paper, the development of ring-like vortices and the mechanism of sustainability of these vortices in the upper boundary layer are investigated numerically by direct numerical simulation (DNS). Intense interaction among ring-like vortices and the other vortical structures in different vortical packets which generated at different time are observed. It shows that steamwise counter-rotating cores will generate new ring-like vortices and the ring-like vortices become more stronger and raise to the upper boundary layer after mutual interaction and merging. In the meanwhile, the boundary layer of the late transition becomes thicker.

Keywords: Evolution; Transition; Vortical structures; Interaction; Filaments flow; Parameters; Simulation; Instability; Downstream; Aggregations; Transitional flow

Introduction

Experimental and numerical results show that when the ring-like vortex structures (hairpin or Ω-shaped vortices) are formed [1-5], the late boundary layer transition begins. Almost at each transitional stage of a boundary layer, these structures are usually found in larger vortex packages. It has also been found that they are the dominant mechanism of perturbation in the late stages of boundary-layer transition.

It is, of course, very important to understand the formation and evolution of Λ vortex structures (especially the ring-like vortices) and the evolution of Λ vortex packages which is associated with the onset of the flow transition. In order to gain a better understanding of the transition process at the late stage, we used a high-order DNS to study its mechanisms in a boundary layer [6-11]. In our previous work [8], we studied the origin and formation of vortex and ring-like vortex numerically. It is found that the widely recognized process, the self-deformation Λ of vortex into a ring-like vortex, does not exist. The so-called hairpin vortex is actually the combination of separated Λ vortex and ring-like vortex. The Λ vortex in the upstream is not a general vortex tube. Indeed, it is a pair of open rotation cores [8,9]. The roots of Λ vortex are formed by special gathering of vortex filaments. On the other hand, ring-like vortices are formed separately and independently. The ring-like vortices and the Λ vortex roots are generated separately by different mechanisms. It is confirmed that the shear layer near the top edge of the boundary is unstable and it will produce such ring-like vortex structures [7].

The purpose of this paper is to further study the mechanism of boundary layer growth during the transition. The evolution of ring-like vortices and vortical packets is studied in detail. In Section II, We briefly introduce the case settings and code validation; in Section III, the vortex structure and its mechanisms from our DNS observation are specified; in Section IV, the evolution process and mechanism of ring-like vortex and vortex package are studied. Finally, we give our conclusions.

Case Setup and Code Validation

Case setup

This paper employs DNS to study the transition process on a flat plate. The grid system we used is 1920 × 128×241, representing the number of grids in the direction of the streamwise (x), the spanwise (y) and the wall normal(z). In the normal direction, the grid is stretched. In the streamwise and spanwise directions, the grids are evenlydistributed. At the inlet, the length of the first grid interval in the normal direction is 0.43 in wall units (y+=0.43). Table 1 lists the flow parameters in our simulation, including Mach number, Reynolds number etc. Here, in x represents the distance between leading edge and inlet, Lx,Ly,Lzin are the lengths of the computational domain in x − , y − , z − and z − directions, respectively, and is the wall temperature.

Code validation

The validations by NASA Langley and UTA researchers of our DNS code can be found in [3-5]. The DNS resultsare well verified by comparison with theoretical and experimental data and are consistent with other DNS results [6-9].

By using the Ω visualization method [12], the vortex structures initialed by the nonlinear evolution of T-S wavesduring the transition are shown in Figure 1. Reference 8-11 give details of the evolution. The formation of ring-likevortex chains coincides with the experimental results [13] and the numerical results of Rist and his co-authors (Bake et al. [14]).

DNS Observations on the Vortical Structures in the Boundary Layer

Figure 1 shows the vortical structure from our numerical simulation at different time steps are visualized by the is surfaceof Ω (Ω= 0.5). At t=6.0T, the Λ vortex in Figure 1a is still open at the tip. However, at t=6.1T, the first ring-likevortex will soon be generated at the tip of the vortex. After that, more ringlike vortices are generated above the Λ vortex when it travels to the downstream (see Figure 1c and 1d).

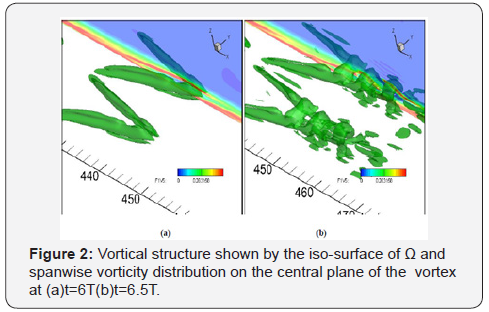
Our previous work [7,8] pointed out that the high shear layer between the roots of Λ vortex (see Figure 2a) is the mechanism by which the ring-like vortices are generated. The high shear is initiated by the input TS waves and formed by rotation effect of the roots of the vortex, especially the ejection which brings the low speed fluid up to approach the high-speed fluids and forms a very strong shear. This very strong shear layer in the middle of Λ-vortex roots is unstable and will break up (see Figure 2b) to several pieces. The ring-like vortices above the Λ-vortex are formed by the breakdown of the shear layer corresponding the Kelvin-Helmholtz instability

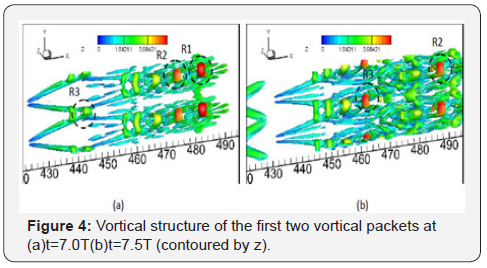
Once the ring-like vortices are formed, the ejecting and sweeping effect [6,9] becomes more intense as we call the second ejection and second sweep. Along with the streamwise vortical structures (such as Λ vortices), low speed zones will be rolled up successively. On the up-bound of these low speed zones, new strong velocity shear layers will be formed (See Figure 3). Most of these velocity shear layers are unstable, and they will also break down to produce more secondary ring-like vortices. At the same time, the streamwise vortical structures will cause more counter-rotating secondary streamwise vortical structures (see Figure 1d). The energy from the free stream and the spanwise vorticity from the lower boundary layer will contentiously trigger or indirectly produce more vortices under the first generated ring-like vortices [9]. The vortical structure soon becomes very complicated. Each vortex will eventually form a vertical packet in the downstream (see Figure 1d and Figure 4). The vertical packets grow and become wide enough to interact with each other (see Figure 4b). The neighboring vortical packets in the direction of spanwise inevitably converge together.

DNS Observations on the Growth of Ring-like Vortices

Figure 5 shows the vortical structure in the boundary layer at t=10T. The ring-like vortices at different levels are continually generated. They dominate the development of the boundary layer, especially the upper boundary layer (see Figure 6).

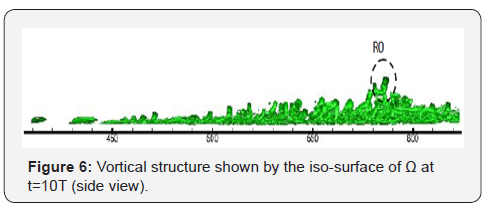
As we know, the tilted ring-like vortices will be raised up by self-induction. In such process, however, the ring-like vortices would be weakened due to dissipation in the upper boundary. Figure 7a shows the change of height of the first and second ringlike vortices in Figure 4 (marked as R1 and R2) when they are traveling to the downstream. These two vortices stop raising up in the downstream. Accordingly, the spanwise vorticity at the tops of them keeps decreasing (see Figure 4b). These ring-like vortices will be dissipated eventually. Thus, there must exist other mechanisms for the sustenance and growth of ring-like vortices at the upper boundary layer (see Figure 5). In order to investigate the mechanisms, evolution of the highest ring-like vortex, marked as R0, in Figure 5 and 6 at t=10T is tracked.

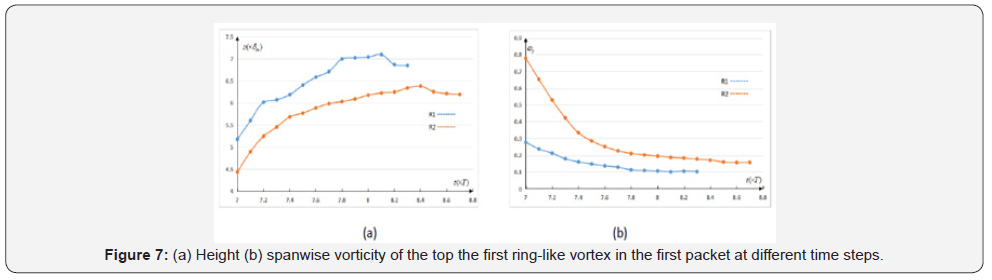
Figure 8 shows the evolution of the vortex R0 in Figure 5 at different time steps. Since the ring-like vortices travel faster than the spanwise vortical structures (such as Λ-vortices) in the lower boundary layer, the vortical packet in the upstream will catch up with the ones in the downstream (see Figure 4b). The vortex R3 in the second packet starts to interact with a new generated ring-like vortex (R4 in Figure 8b) in the first packet at t=8.5T. Eventually, these two ring-like vortex merges together and become ring-like vortex R0 in Figure 5.

Figure 9 gives the spanwise distribution on the central streamwise plane in the process of forming the large ring-like vortex R0. The tops of the vortex R3 and R4 are circled. It can be seen clearly that the intensity of the ring-like vortex R3 is reduced a lot before it catches the first vortical packet. However, it will be strengthened after entering into the first vortical packet, especially when the interaction happens with R4 - a new generated ring-like vortex in the downstream. The merge of these ring-like vortices creates a larger and much stronger ringlike vortex-R0 (Figure 9c).

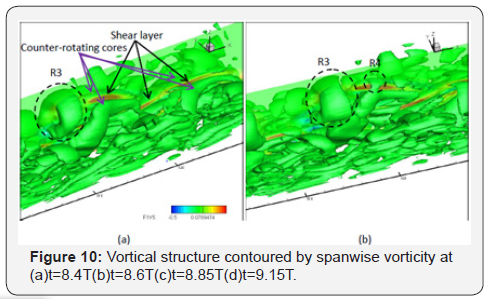
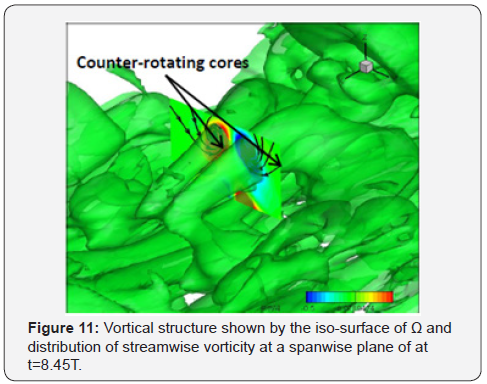
Figure 10 shows the vortical structure in the downstream of the vortex R3 and the distribution of spanwise vorticity on the central plane. It shows that the newly generated ring-like vortex R4 at t=8.55T is generated at the upper bound of a shear layer by the instability of this shear-layer (K-H instability). The shear layer is created by the up-wash effect of the counter-rotating cores (see Figure 10 and 11). This kind of counter-rotating cores are a common vortical structure in the transitional flow (see Figure 4b, 8d and10a). They are not vortex tubes but still the aggregations of vortical filaments which are similar to Λ-vortices [8]. Strong shear layers are continually formed between these counter-rotating cores and new generations of ring-like vortices will happen at the upper bounds due to K-H instability (see Figure 10). The existence of streamwise counter-rotating cores and the shear layers is one of the mechanisms for generating and sustaining ring-like vortices in the upper boundary layer in the transitional flow.

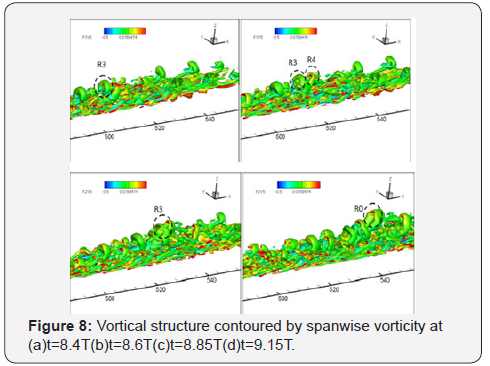
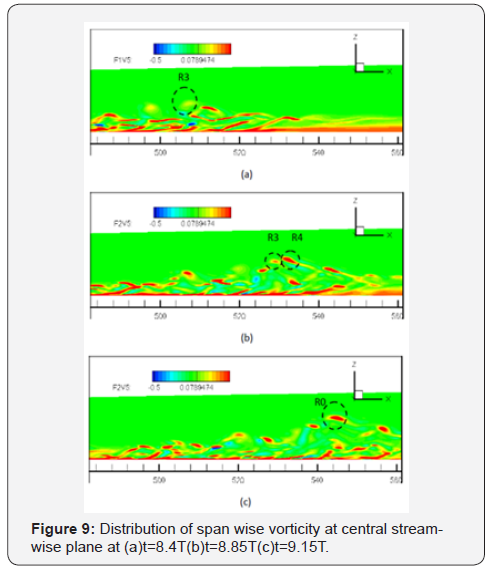
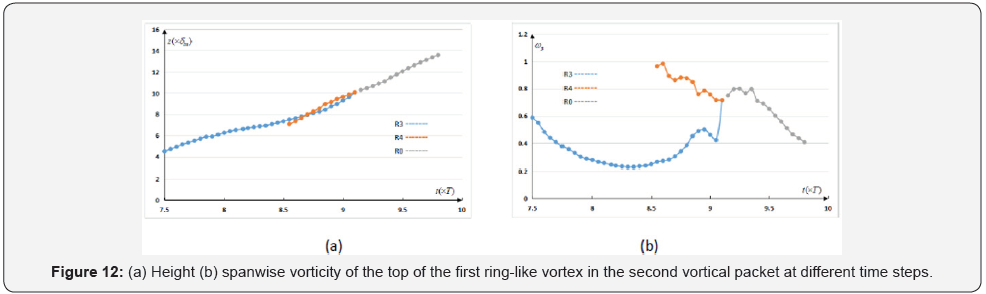
Figure 12a gives the heights of the vortex R3 from 7.5T to 9.7T. It shows that this ring-like vortex keeps rising. The height of this ring-like vortex is lower than the vortices R1 and R2 in the first vortical packet at t=7.5T (also see Figure 4). However, it reaches the height of 13.3δin which is twice as the maximum height of R1 has ever reached. It must be pointed out that, the increase of the height of R3 seems linearly, even after it caught the first vortical packet. There’s no jump on the height after the second vortical packet mixed with the first packet. When two vortical packets meet together, the second vortical packet is not simply pushed up. Complicated interaction among vortices and other vortical structures will happen.

In Figure 12b, the spanwise vorticity at the top of the ringlike vortex R3 and R4 is provided. It shows that the ring-like vortex R3 is first reduced before it catches the first vortical packet. Then, the intensity of its top become increasing and oscillating. The vorticity increases and reaches a peak when R3 merges with R4 (become R0) at about t=9.1T. Of course, when the new generated ring-like vortex R0 keeps arising, it will be reduced again (see Figure 12b).

During the evolution of vortical structures in the downstream, more and more different vortical packets are mixed, some of the ring-like vortices will continually be strengthened by mutually interaction and merging. In the meanwhile, more secondary ring-like vortices and other vortical structures will be created. The boundary layer is thickened gradually during this process. At last, the typical forest of vortical structure will be formed in the boundary layer (see Figure 5). The interaction between vortical packets are very complicated and require further studied in detail.

Conclusion

In this paper, the study on the development of ring-like vortices in the transitional flow by DNS (direct numerical simulation) is given. This includes the mechanisms of the formation of large ring-like vortices in the upper boundary layer, the mutual interaction among ring-like vortical structures and the increase of boundary layer thickness. It is found that the streamwise counter-rotating cores played a very important role on generating new ring-like vortices in the upper boundary layer. The ring-like vortical structures generated in different time and different packets also interacts intensely. Once the vortical packets meet together, the ring-like vortices will interact and merge with each other. The strengthened ring-like vortical structures will move towards a higher position and become stronger. The boundary layer thus becomes thicker gradually during the process.

Acknowledgment

The authors are grateful to Texas Advanced Computing Center (TACC) for providing computation hours. This work was partially supported by Science and Technology Commission of Shanghai Municipality (Grant No. 13DZ2260900). This work is accomplished by using Code DNSUTA which was released by Dr. Chaoqun Liu at University of Texas at Arlington in 2009.

References

1. Hama FR (1960) Boundary-layer transition induced by a vibrating ribbon on a flat plate. Proc. Heat Transfer & Fluid Mech. Inst. (Palo Alto, Calif: Stanford Univ. Press) pp. 92-105.
2. Hama FR, Nutant J (1963) Detailed flow-field observations in the transition process in a thick boundary layer. Proc. Heat Transfer & Fluid Mech. Inst. (Palo Alto, Calif.: Stanford Univ. Press) pp. 77-93.
3. Liu C, Liu Z (1995) Multigrid mapping and box relaxation for simulation of the whole process of flow transition in 3-D boundary layers, J. of Computational Physics 119(2): 325-341.
4. Chaoqun L, Zhining L (1997) Direct Numerical Simulation for Flow Transition Around Airfoils, Proceedings of First AFOSR International Conference on DNS/LES, Louisiana Tech University, Ruston, Louisiana, pp. 13-28.
5. Zhining L, Guohua x, Chaoqun L (1996) Direct numerical simulation for the whole process of transition on 3-Dairfoils.AIAA paper, USA.
6. Liu C, Chen L, Lu P, Liu X (2010) Study on Multiple Ring-Like Vortex Formation and Small Vortex Generation in Late Flow Transition on a Flat Plate, Theoretical and Numerical Fluid Dynamics.
7. Yonghua Y, Chaoqun L (2013) Shear Layer Stability Analysis in Boundary Layer Transition and MVG Controlled Ramp Flow. AIAA paper.
8. Yonghua Y, Caixia C, Huankun F, Chaoqun L (2014) DNS Study on λ-Vortex and Vortex Ring Formation in Flow TransitionJournal of Turbulence 15(1): 1-21.
9. Liu C, Yan Y, Lu P (2014) Physics of turbulence generation and sustenance in a boundary layer, Computers & Fluids 102: 353–384.
10. Yonghua Y, Caixia C, Huankun F, Chaoqun L (2013) DNS Study on Lambda Vortex and Vortex Ring Formation by Vortex Filaments in Flow Transition. AIAA paper.
11. Yan Y, Tang J, Liu C, Yang F (2016) DNS Study on the Formation of Lambda Rotational Core and the Role of TS Wave in Boundary Layer Transition. Journal of Turbulence17(6): 572-601.
12. Liu C, Wang Y, Yang Y, Duan Z (2016) New omega vortex identification method, SCIENCE CHINA Physics, Mechanics & Astronomy 59(8): 684711.
13. Guo H, Borodulin VI, Kachanov YS, Wang JJ, Lian QX, et al. (2010) Nature of sweep and ejection events in transitional and turbulent boundary layers. Journal of Turbulence 11.
14. Bake S, Meyer D, Rist U (2002) Turbulence mechanism in Klebanoff transition:a quantitative comparison of experiment and direct numerial simulation. J.Fluid Mech 459: 217-243.