Open AccessJournals | List of Open Access Journals

Research Article

Determination of Natural Frequencies of Arch Dams Based on Reservoir Levels Using Ambient and Forced Vibration Tests

Bariş Sevim¹ and Ahmet Can Altunişik²*

¹Department of Civil Engineering, Yildiz Technical University, Istanbul, Turkey

²Department of Civil Engineering, Karadeniz Technical University, Trabzon, Turkey

Submission: December 05, 2017; Published: March 13, 2018

*Corresponding author: Ahmet Can Altunijik, Assoc. Prof, Department of Civil Engineering, Karadeniz Technical University, Trabzon, Turkey, Tel : + 90462 3774020; Email: [email protected]

How to cite this article: Bariş S, Ahmet C A.Determination of Natural Frequencies of Arch Dams Based on Reservoir Levels Using Ambient and Forced Vibration Tests. Civil Eng Res J. 2018; 3(5): 555623.DOI:10.19080/CERJ.2018.03.555623

Abstract

In this study, the dynamic characteristics such as natural frequencies of arch dams are investigated using Ambient and Forced Vibration Tests. For this purpose, a prototype model of Type-1 arch dam considering reservoir and foundation is constructed in the laboratory conditions. The dam has a single curvature, constant radius and constant central angle. Also, it has 6 unit height and 0.6 unit constant widths. To construct a prototype model, 1 unit is selected as 10cm. Therefore, dam height and crest width are designed as 60cm and 6cm, respectively. To identify dynamic characteristics of the arch dam, ambient and forced vibration tests are conducted for different reservoir levels; and the variation of natural frequencies is determined using both Ambient and Forced Vibration Tests. During the ambient vibration tests the dam vibrated under natural excitations in operation conditions. During the forced vibration tests, the dam excited by an impact hammer. From the study, the dynamic characteristics obtained for different reservoir level are compared with each other. It is seen that there is a good agreement between both results and they are nearly closed to each other. Also, the higher reservoir levels significantly change the behavior of the dam.

Keywords: Ambient vibration test; Dynamic characteristics; Forced vibration test; Natural Frequency; Prototype arch dam model; Reservoir level

Abbreviations: FRF: Frequency Response Function; IRF: Impulse Response Function; PP: Peak Picking; SSI: Stochastic Subspace Identification; FDD: Frequency Domain Decomposition techniques; EFDD: Enhanced Frequency Domain Decomposition; SVD: Singular Value Decomposition; FFT: Fast Fourier Transform

Introduction

Arch dams generally curved in plan and possibly in elevation, and they transmits a huge portion of the water pressure and other loads such as earthquake, wind, ice etc. [1]. They are required advanced engineering knowledge to design, construct and manage. Although arch dams have multiple benefits, they present a risk to public safety due to the possibility of a dam failure and the damage it would cause [2]. Especially, the damage of these dams retaining large quantities of reservoir include serious risks for life and property during earthquakes. To prevent these risks, dynamic behavior of the structure have to be assessed. Ambient vibration Testing (AVT) and Forced Vibration testing (FVT) are the useful tools to determine the dynamic properties of structures. The importance of the subject is highligted in some studies [3-7]. In vibration testing dynamic behavior of a structure is estimated through a number of natural frequencies and mode shapes [8]. Although at a low level of excitation this experimental procedure is very useful to validate the numerical results used to evaluate the seismic behavior of dams [7].

Last two decade, some researchers have investigated to dynamic behavior of large dams using both ambient and forced vibration testing methods. In these studies it is highlighted that although the physical conditions are hard as difficulties to excite of large dams, and test become too expensive; results obtained AVT and FVT are very attractive and useful [9-13]. Such kind of conditions made to researchers to construct scaled prototype dam models to study experimentally [14-18]. However, when constructing scaled dam models it is very important to take great care in due to scaled models represent the real dam.

In this study, it is investigated that reservoir level effects on the dynamic characteristics of a prototype arch dam model using AVT and FVT. For this purpose, a prototype concrete arch dam- reservoir-foundation model is constructed in the laboratory conditions. Ambient and forced vibration tests are conducted to the arch dam to identify dynamic characteristics for different reservoir levels, and the variation of natural frequencies is determined using both methods. The results showed that natural frequencies obtained for different reservoir level from both methods are near to each other. It is highlighted in the study that both of methods are very useful to determine the dynamic behavior of arch dams.

Main Principles of Ambient and Forced Vibration Testings

Ambient vibration testing

Ambient excitation does not lend itself to Frequency Response Function (FRFs) or Impulse Response Function (IRFs) calculations because the input force is not measured in an ambient vibration test. Therefore, a modal identification procedure will need to base itself on output-only data [19]. There are several modal parameter identification techniques available such as Peak Picking (PP), Stochastic Subspace Identification (SSI), and Frequency Domain Decomposition techniques (FDD). These techniques are developed by improvements in computing capacity and signal processing procedures. In this study Enhanced Frequency Domain Decomposition technique (EFDD) is used to extract dynamic characteristics of the arch dam in OMA.

EFDD technique is an extension to FDD technique. FDD is a basic technique that is extremely easy to use. In the technique, modes are simply picked locating the peaks in Singular Value Decomposition plots (SVD) calculated from the spectral density spectra of the responses. As FDD technique is based on using a single frequency line from the Fast Fourier Transform analysis (FFT), the accuracy of the estimated natural frequency depends on the FFT resolution and no modal damping is calculated. Compared to FDD, EFDD gives an improved estimate of both the natural frequencies and the mode shapes and also includes damping [20].

In FDD technique, the relationship between the unknown input x (t) and the measured responses y (t) can be expressed as [21]:

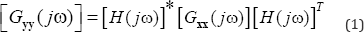
Where G_xx(jw)is the Power Spectral Density (PSD) matrix of the input, r is the number of inputs,G_yy(jw) is the mxm PSD matrix of the responses, m is the number of responses, H(jw) is the Frequency Response Function (FRF) matrix, and and *superscript T denote complex conjugate and transpose, respectively. Solution of the Equation (1) is given detail in the literature [22].

Forced vibration testing

The Frequency Response Function (FRF) is a fundamental measurement that isolates the inherent dynamic properties of a structure. Natural frequencies, mode shapes and damping ratios are obtained from a set of FRF measurements. The FRF describes the input-output relationship between two points on a structure as a function of frequency. Since both force and motion are vector quantities, they have directions associated with them. Therefore, an FRF is actually defined between a single input DOF (point &direction), and a single output DOF [23,24].

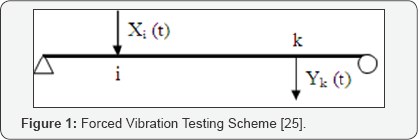
With Forced Vibration Testing the structure is artificially excited with a forcing function in a point i and its response y_k (t) to this excitation is measured together with the forcing signal x_k (t) (Figure 1). Transformation of these time signals into the frequency domain and calculation of all Frequency Response Functions (FRF) H_ik between the response and the forcing function time signals yields the Frequency Response Matrix, also referred to as Transfer Matrix, H(iw) (Figure 2) [25].

In this study, FRF measurements of the arch dam are obtained from impact testing. Because impact testing is a one of the fast, convenient, and low cost way of finding the modes of structures. Also, in small and medium size structures, the excitation can be induced by an impulse hammer. But the selection of the hammer type can have a significant effect on the measurement acquired. The input excitation frequency range is controlled mainly by the hardness of the type selected [26]. The schematic view of the impact testing is shown in Figure 3.

Experimental Example

Description of type-1 arch dam

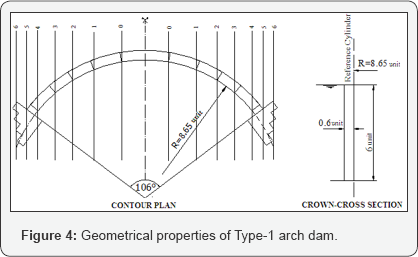
In this study, Type-1 arch dam suggested "Arch Dams" Symposium in England in 1968 [27], is selected for the experimental example. Type-1 arch dam has the simplest geometry presented of the symposium. The geometrical properties of Type-1 arch dam are shown Figure 4. As it is seen from Figure 4, Type-1 arch dam has a single curvature, constant radius and constant central angle. In addition, it has 6 unit height and 0.6 unit constant widths. To construct a prototype model, 1 unit is selected as 10cm. Therefore, dam height and crest width are designed as 60cm and 6cm, respectively. 3D finite element model of Type-1 arch dam is constituted by ANSYS [28] software (Figure 5).

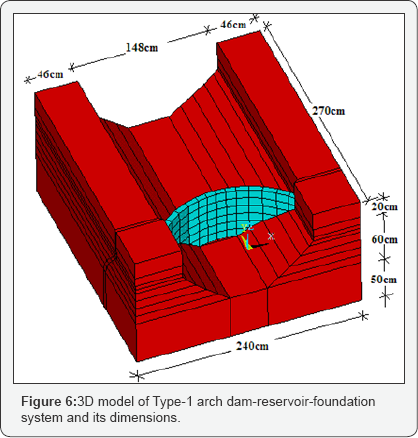
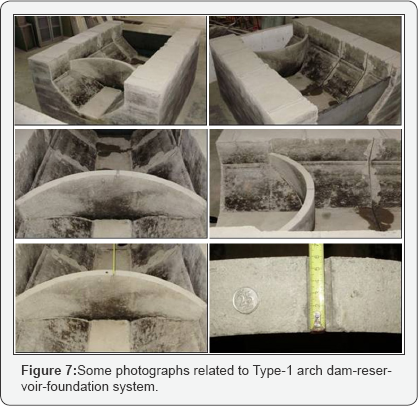
Type-1 arch dam is developed including reservoir and foundation. The reservoir is extended as 3H (H: dam height); the foundation is extended as about H in the downstream and downward directions, and is extended as reservoir length in upstream direction. Such a modeling is appropriate to represent the dynamic response of concrete dams [2]. Definitive dimensions of Type-1 arch dam-reservoir-foundation system are shown in Figure 6. Type-1 arch dam-reservoir-foundation system is constructed considering dimensions given in Figure 6. Some photographs related to dam are shown in Figure 7.

Ambient and forced vibration tests

Ambient and forced vibration tests are conducted to Type- 1arch dam model to determine its natural frequencies, mode shapes and damping ratios. Measurements are implemented for empty reservoir, 10cm, 20cm, 30cm, 40cm, 50cm reservoir levels and full reservoir.

The selection of measurement equipment, and measurement setup such as measurement time and frequency span are so important in the vibration tests. Because, measurement equipment and setup are variable considering structure type. In the both vibration tests, B&K 3560 data acquisition system with 17 channels and B&K 4507-B005 type uni-axial accelerometers are used. These accelerometers have 1000mV/g sensitivity and 0.1-6000Hz frequency span. During the tests, frequency span is selected 0-1600Hz according to finite element results. Also B&K 8206-002 type impact hammer with 2.27mV/N sensitivity and 4500N maximum force is used during the FVT.

During the AVT, measurements are performed along two minutes, and excitations are provided from small impact effects. Signals obtained from the tests are recorded and processed by the commercially softwares PULSE [29] and OMA [30]. Dynamic characteristics of Type-1 arch dam are determined by EFDD technique existed in OMA software. During the FVT, linear averaging method is selected during the tests and number of the samples is selected 5.FRFs of the measurements are obtained from PULSE software. In the forced vibration tests, only the natural frequencies are determined due to optional tools are not found in the test equipment to determine mode shapes and damping ratios.

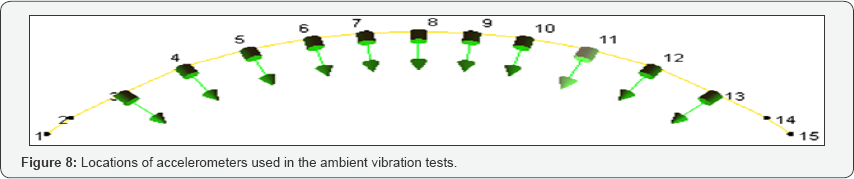
Ambient vibration tests and dynamic characteristics of type-1 arch dam: Ambient vibration tests are conducted to dam for empty reservoir, 10cm, 20cm, 30cm, 40cm, 50cmreservoir levels and full reservoir. During the tests, eleven accelerometers are located to normal direction of the water in the local axial along to crest of the dam (Figure 8). Some photographs from the tests related to each reservoir level are shown in Figure 9.

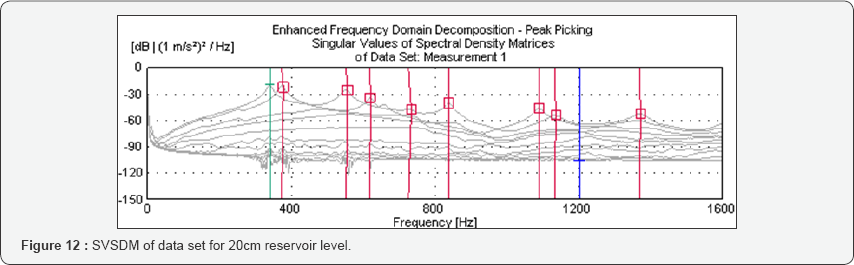
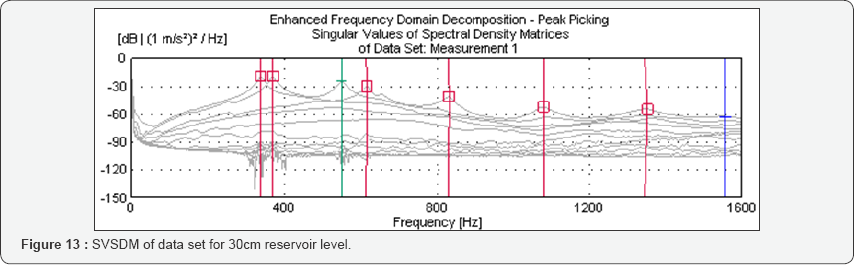
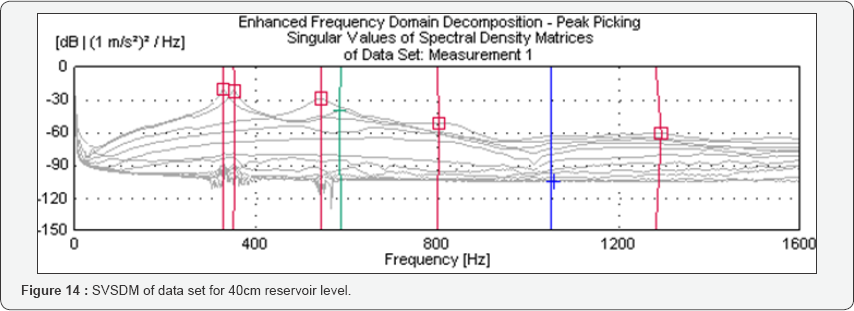
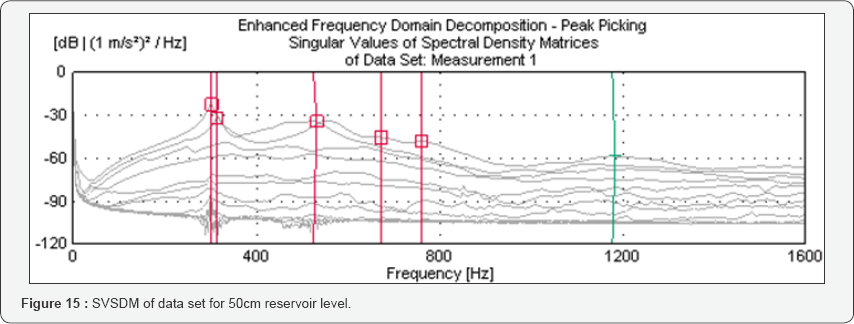
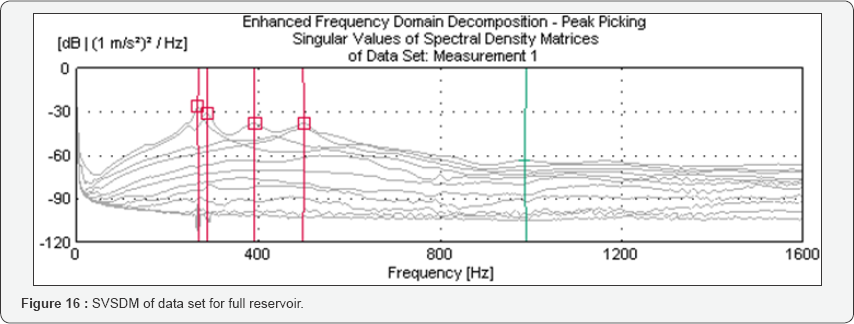
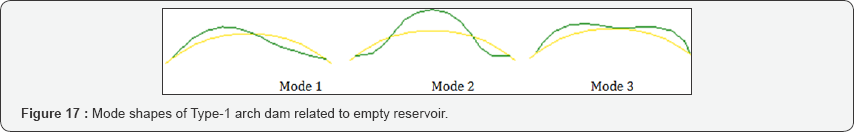
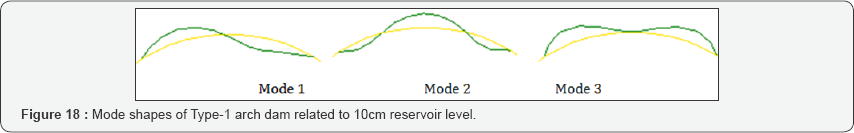
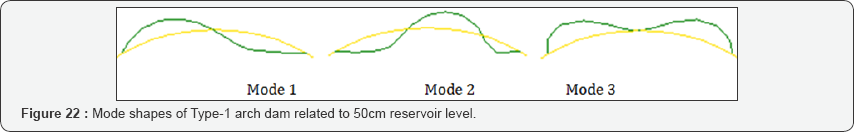
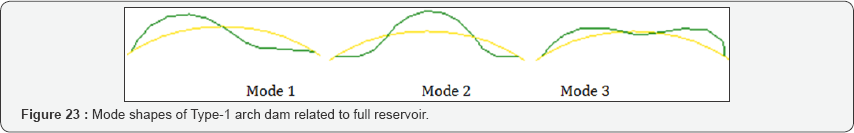
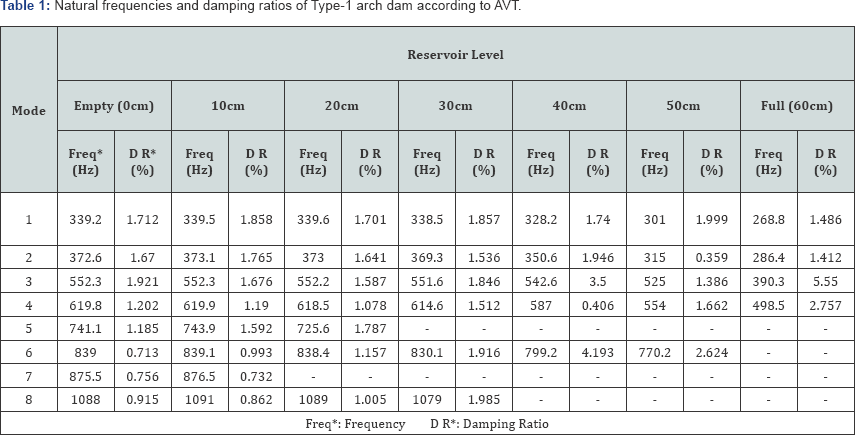
Singular values of spectral density matrices (SVSDM) of data set are shown in Figures 10-16 for each reservoir level, respectively. Mode shapes obtained from the tests for each reservoir level are shown in Figures 17-23. Due to locations of the accelerometers is along to normal direction of the water, only symmetrical and anti-symmetrical modes are obtained. The natural frequencies and damping ratios obtained from the tests are listed in Table 1. Natural frequencies are not overly changed related to each mode shape among 0-30cm reservoir levels, however the shift is overly increased after 30cm. Also natural frequencies are reduced for the high reservoir levels.

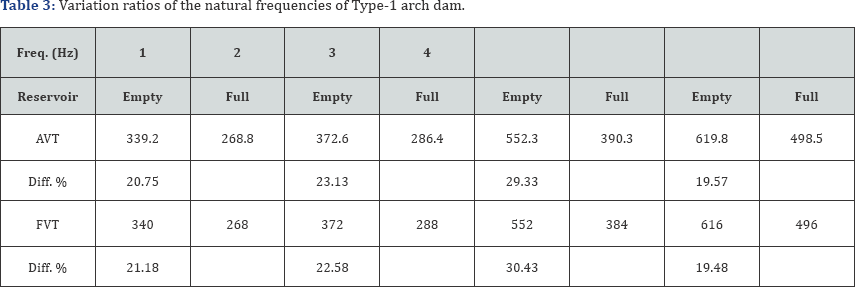
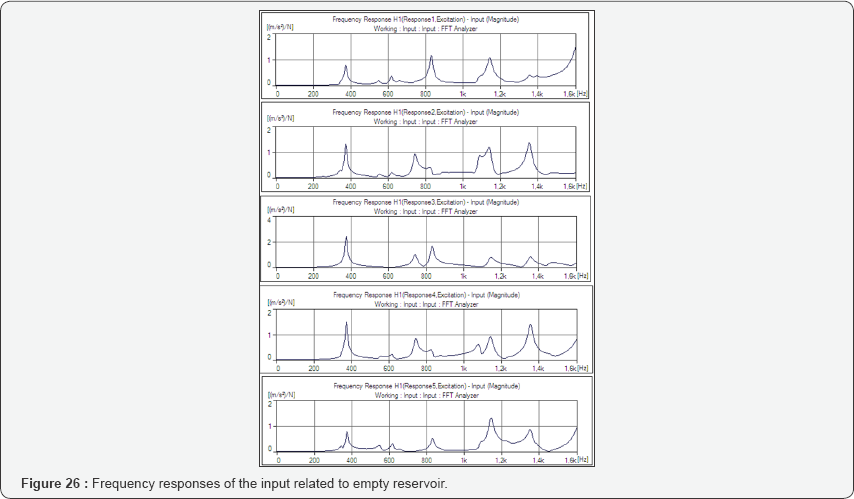
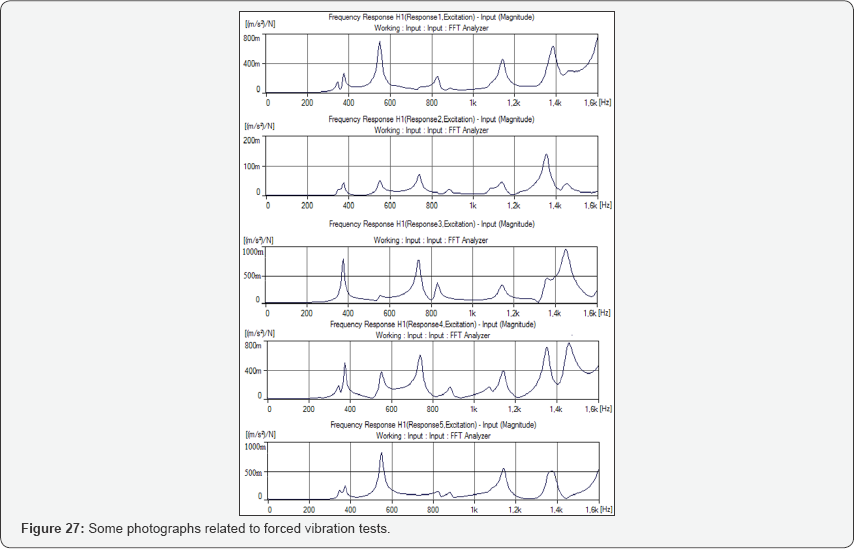
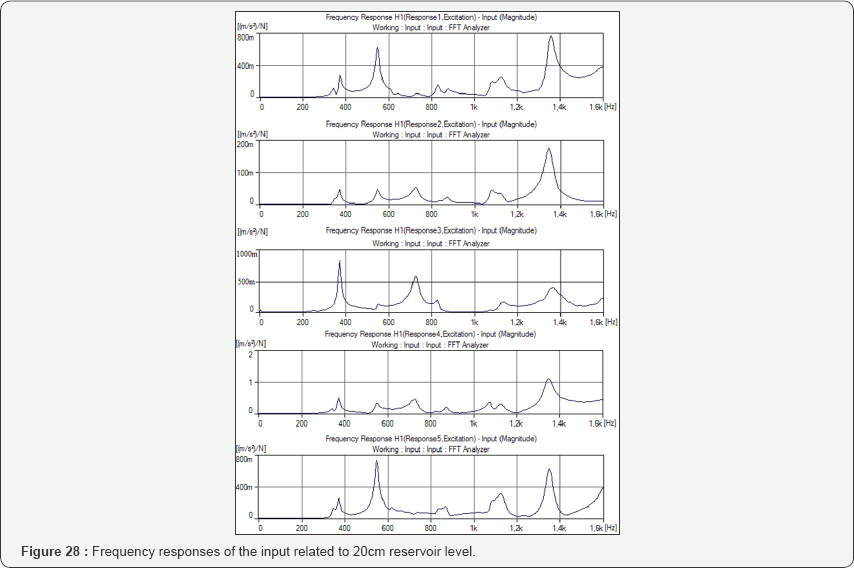
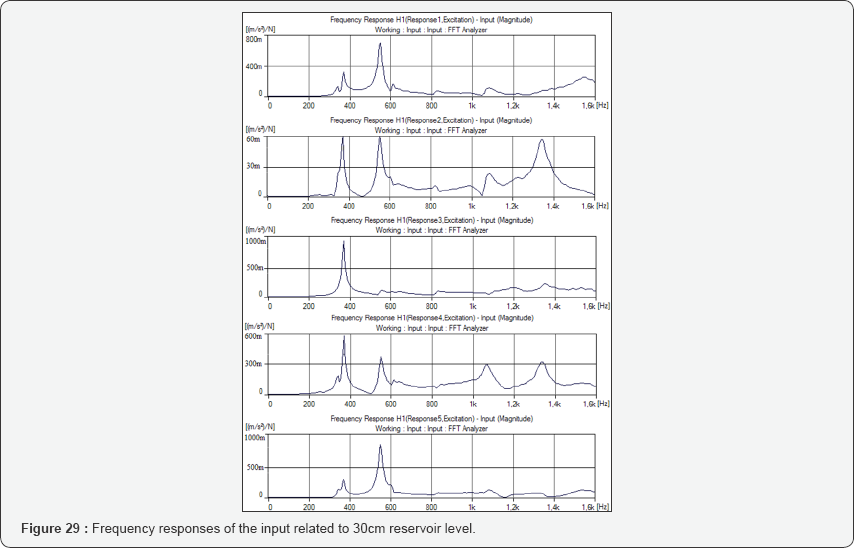
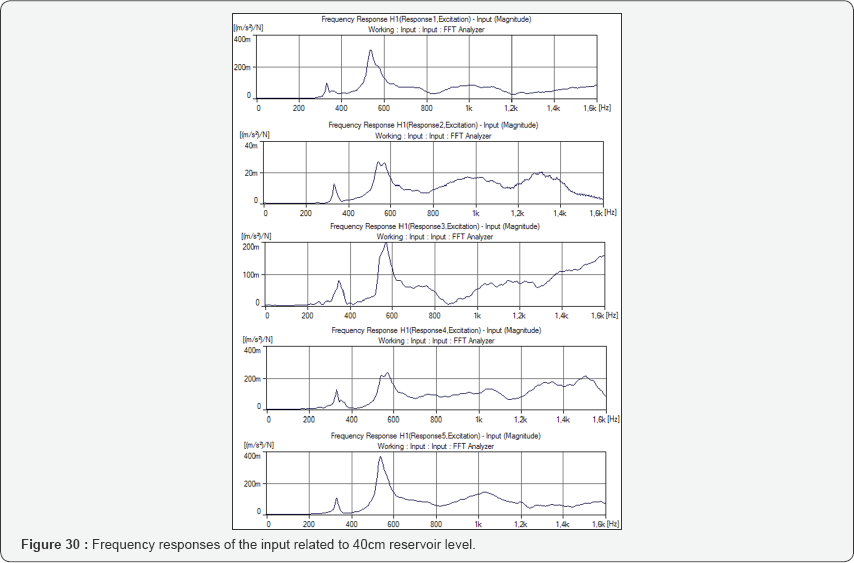
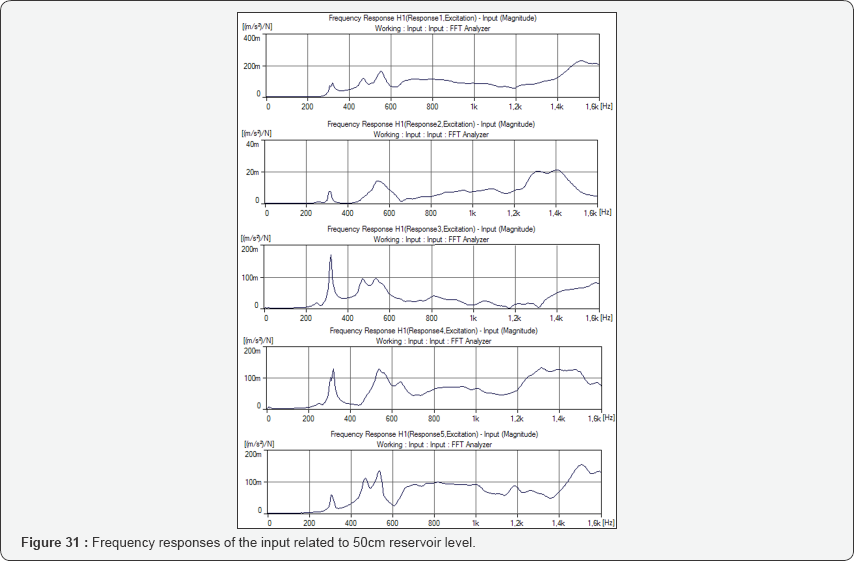
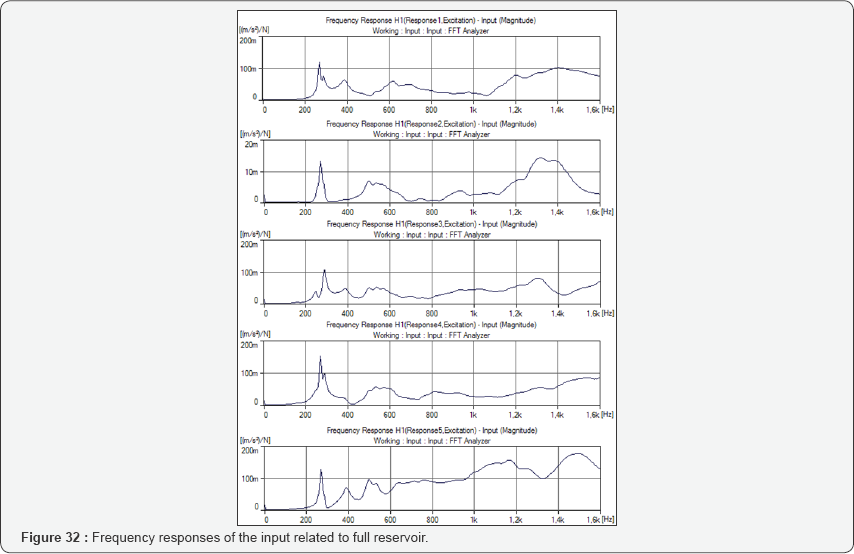
Forced vibration tests and natural frequencies of type- 1 arch dam: Forced vibration tests are conducted to dam for the same reservoir levels measured in AVT. During the tests, five accelerometers are located to normal direction of the water in the local axial along to crest of the dam (Figure 24). Some photographs related to each reservoir levels are shown in Figure 25. Frequency responses of the inputs are shown in Figures 26- 32. The natural frequencies of Type-1 arch dam are listed in Table 2.

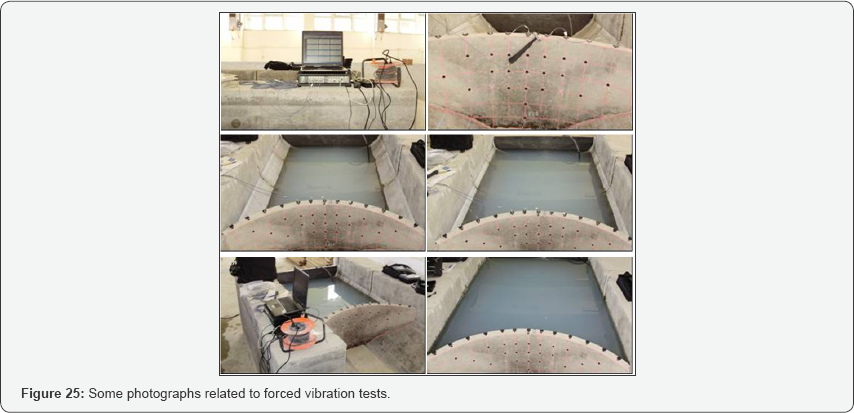
Variation of natural frequencies of type-1 arch dam: Variation of the first four natural frequencies obtained from AVT and FVT are plotted in Figure 33a-33d, respectively. As it is seen Figure 33, the natural frequencies of Type-1 arch dam are not overly changed among 0-30cm reservoir levels. For the higher levels from half of dam height, the natural frequencies are overly reduced. The variation ratios of the natural frequencies for the empty and full reservoirs are given in Table 3.

Conclusion

In this study, the reservoir level effects on the dynamic characteristics of arch dams are investigated using Ambient and Forced Vibration Testings. For this purpose, a prototype model of Type-1 arch dam considering reservoir and foundation is constructed in the laboratory conditions. To identify dynamic characteristics of the arch dam, ambient and forced vibration tests are conducted for different reservoir levels; and the variation of natural frequencies is determined using both ambient and forced vibration tests. The following observations can be made from the study:

o The natural frequencies for each reservoir level obtained from AVT and FVT are almost close to each other. Only, the frequencies obtained for 50cm reservoir level are different

o The natural frequencies of Type-1 arch dam are not overly changed among 0-30cm reservoir levels. But, for the higher levels from half of dam height, the natural frequencies are overly reduced.

o It is difficult to obtain modes with the increasing reservoir level. Although 8-9 modes can be obtained for the lower reservoir levels, only 3-4 modes can be obtained for higher reservoir levels. It can be stated that the higher reservoir levels complicate the dynamic behavior of Type-1 arch dam.

o The first four natural frequencies of Type-1 arch dam with empty and full reservoir are changed 20-25 % approximately.

Acknowledgement

This research was supported by TUBITAK and Karadeniz Technical University under Research Grant No. 106M038, 2006.112.001.1. The authors thank to KTU Hydraulic Lab. workers.