Open AccessJournals | List of Open Access Journals

Research Article

Reliability of Optimized Peak Power in Swimming

Emma Swanwick* and Martyn Matthews

School of Health Sciences, University of Salford, UK

Submission: February 28, 2018; Published: March 13, 2018

*Corresponding author: Emma Swanwick, School of Health Sciences, University of Salford, UK; Email: ek.swanwick@gmail.com

How to cite this article: Emma Swanwick, Martyn Matthews. Reliability of Optimized Peak Power in Swimming. J Phy Fit Treatment & Sports. 2018; 2(2): 555584 DOI: 10.19080/JPFMTS.2018.02.555584

Abstract

Testing of power in swimming has previously demonstrated values that are far below those seen in other sports with similar level subjects. The purpose of the study was to evaluate the use of a modified optimized peak power (OPP) test in swimming. 10 swimmers swam 4 bouts of 15m from a rolling start at maximal effort on two occasions. Each time, the swimmer was attached to the Power Reel (Total Performance Inc, Ohio USA) at resistances of 3%, 5.5%, 7.5% and 8.5% of body mass applied in random order. Swim times increased significantly as resistance rose in both test 1 & 2 except for between swim 3 & 4 in test 2. There was no significant difference between times (p = 0.11- 0.43), stroke rate (p = 0.27-0.48) or OPP (876.4 ± 117.9W) vs (884.7 ± 104.7W) r² =0.95 p = 0.18. The values achieved by swimmers using the OPP are more in line with values seen in athletes of similar level in other sports. We conclude that the OPP swim test is a more appropriate method for testing power in swimming.

Introduction

In the last 30 years there have been a number of attempts to measure and evaluate propulsive force in swimming. Tethered swimming has remained a favorite for many years [1-5]. Other alternatives of testing have been used but can take several hours to complete, analyze and give feedback. Testing of high- performance sportsmen requires tests to be reliable, objective, valid, technology-friendly, and situational-specific as well [6]. The Wingate anaerobic test (WAnT) has been used for gathering the majority of data on peak power (PP) and has been used to identify upper and lower body power relationships to 50m swim speed [7]. The fixed breaking force used in the WAnT does not necessarily optimize power output, particularly in children and adolescents [8]. For this reason, the Optimized peak power test (OPP) was devised [9]. This force-velocity protocol uses a series of brief maximal effort sprints against a range of breaking forces to create a parabolic force-power relationship, from which optimal peak power, optimal velocity and optimal breaking force can be calculated [8].

Testing swimmers in the water, however, offers a range of difficulties such as how to apply a set force, whether in relation to body weight or to the residual weight not buoyed by the water. Fixed swimming changes the effort created in the body to attempt to overcome the fixed force [10], whereas free swimming does not allow for OPP to be determined. Additionally, applying a load to the body requires the load to remain outside of the wake, or the load generated will be reduced by water disturbance [11]. As power is dependent upon the length and volume of the muscle mass being used to generate power, the assessment of lean body mass is essential to identify real power contributions. The effect of buoyancy will also change dependent upon the thickness of skin folds (representing the underlying fat content). Being lean is not always a prerequisite to swimming fast [12-14] as a body that cannot be buoyed sufficiently will require additional energy to retain an optimal position in the water from which to derive drive and swimming speed. OPP has a particular interest for swimming as most power tests using swimmers have either been done on dry land (Bike; Bio-kinetic swim bench) while those carried out in the water [15-17] provide results that would not agree with similar levels of athletes when they are tested specifically. For this reason, using a modified optimized power test that has potential to identify specific power of swimmers in the water offers a major advantage over what has been done previously. To our knowledge, the OPP has not been used in swimming previously.

Methods

Ten members of the Australian Institute of Sport Swimming Team volunteered to participate in this study (age 19.70 ± 0.95, Mass 79.93 ± 5.26). Participants had returned from a 3-week vacation and the experiment was carried out after they had completed 1 week of basic, mixed stroke, training. The AIS research ethics board approved this study. All participants provided both verbal and written confirmation of their consent to participate in the study. The study also adhered to the ethical standards of the Australian sports commission.

Experimental Design

We used a Power Reel (manufactured by Total Performance Inc, Ohio) to apply set loads against swimmers. This is an electrically braked device that can apply specific loads of resistance as set by the operator. The Power Real uses a low tensile steel cable (3mm diameter) and attaches to the swimmer via a belt that fixes around the waist. The cable then trails behind the swimmer without impeding the kick action. Each time before using the Power Reel, we calibrated the machine, by attaching known resistances to the cable and dropping them over a non- resistant bar to drop a distance of 1m and read on the force output of the Power Reel. All tests were carried out in a 25m pool. Swimmers were to swim 15m at maximum effort, from a rolling start. To do this they were allowed to push off the wall but needed to break the water and start swimming just before the 5m flags. They then continued to swim maximally until they reached the flags at the opposite end of the 25m pool (flags at 20m).

This gave the 15m distance required for the test. 15 metres should take elite swimmers between 8 and 12 seconds, dependent upon stroke used. Swimmers started on a set signal, at which two experienced timekeepers started timing the swim, the first time taken as the swimmer crossed the 5m line, (time 1) the second time taken as the swimmer crossed the 20m line (time 2). Time 1 was subtracted from time 2 to give the swimmers 15m time. Each participant attended two testing sessions on separate days. This was to allow comparison and determine the reliability of the OPP test. On each test day, each subject warmed up for 20 minutes using a standardized procedure, including general swimming kicking arms only swimming and some short (15m) acceleration swims. Each subject was then allowed 2 "practice efforts" to get the feel of the resistance. Following the protocols of Winter et al 1995, the resistance for each subject was set at 3%, 5.5%, 7.5% and 8.5% of body mass. In test 1, each subject started at 5.5%BM resistance and the other swim resistances were given in random order. This order was then followed in test 2. As well as time, Stroke count and stroke rate were taken on each swim.

Results

Swim Time

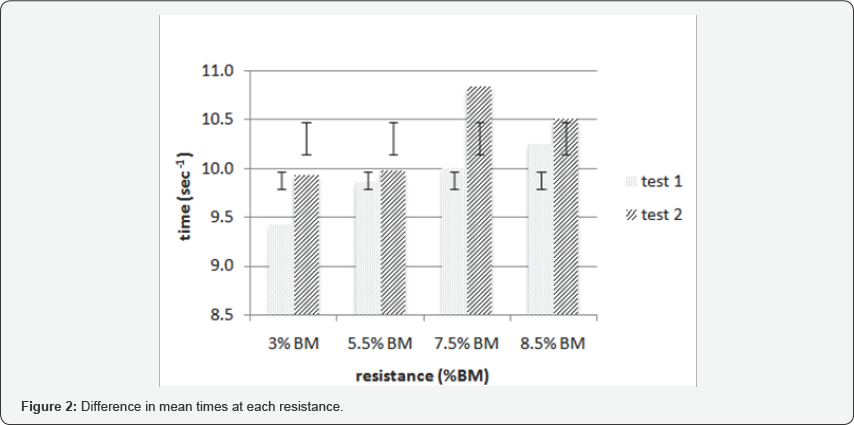
In test 1, the times that swimmers completed the 15m in swim 1-4 were significantly different in all combinations (time 1 vs time 2, time 1 vs time 3, time 1 vs time 4, time 2 vs time 3 time 2 vs time 4 and time 3 vs time 4) p=0.001 and Cohen's effect size was trivial in all tests. In test 2 times were significantly different in all comparisons (p=0.01 effect size, D, was large), except time 3 vs time 4 (p=0.17, Effect size was small). There was no significant difference between swim times in test 1 and test 2 at any resistance (at 3%BM (p=0.20 effect size, cohen's D = Large, at 5.5%BM p=0.43, D = small at 7.5%BM p=0.11, D = Large, at 8.5%BM p=0.18 D = large).

Stroke Rate

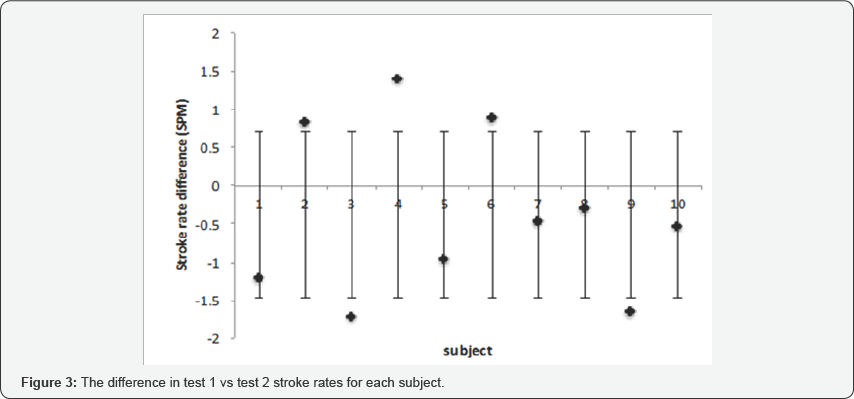
In swim test 1 there was significant difference between stroke rate of swim 1 and swim 2 (p=0.003, D = small), swim 1 and swim 3, (p= 0.009, D= small) and swim 1 and swim 4 (p=0.004, D = large). Additionally, swim 2 was significantly different from swim 4 (p=0.003, D = small) but not swim 3 (p=0.02, D = small). Swim 3 and swim 4 were not significantly different (p=0.18, D = trivial). In test 2 swim 1 was not significantly different from swim 2, (effect size = -.96) and swim 3 was not significantly different from swim 4 (effect size = trivial), but all other combinations were significantly different. (1vs 3 p=0.009, D = medium, 1 vs 4 p=0.004, D = large, 2 vs 3 p=0.01, D = small, 2 vs 4 p=0.001, D = Medium). When the stroke rate in swim one, test 1 was compared to swim 1 in test 2, there was no significant difference (p=0.4). Similarly, no significant difference was found between stroke rate in the 2nd (p = 0.27), 3rd (p = 0.48) and 4th swims 9p = 0.43). Effect size was trivial in all cases.

Power

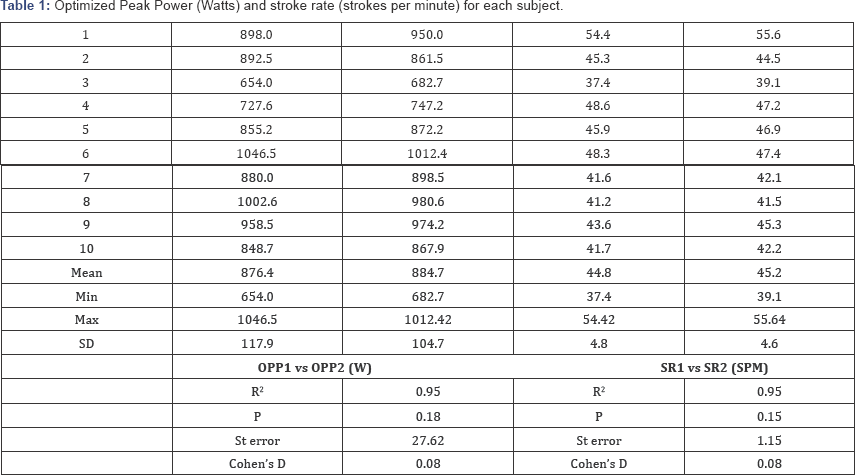
Mean, minimum, maximum power and SD in watts for test 1 and test 2 are shown in Table 1. All comparisons of swim power steps (swims 1 to 2, 1 to 3 1 to 4, 2 to 3, 2 to 4, and 3 to 4 in test 1 and in test 2) were significantly different from each other. When swim 1 in test 1 was compared to swim 1 in test 2 was not significantly difference and effect size was trivial (p = 0.36, D = 0.10). Similarly there was no significant difference and trivial effect size for swims 2, (p=0.24, D = 0.12) 3, (p=0.26, D = 0.02) and 4 (p=0.39, D = 0.06).

Calculated Peak Power

The results of Optimized Peak Power (OPP) and stroke rate for each swimmer, in each test are shown in Table 1. OPP was not significantly different between test one and test two (p = 0.16 r² = 0. 95, effect size was trivial). The relationship between OPP in test 1 and test 2 is shown in Figure 1. The relationship between Swim time and resistance for test 1 and test 2 is shown in Figure 2. Standard deviation for test 1 was 0.25 sec in test 1 and 0.34 sec in test 2. Figure 3 shows the difference between the predicted stroke rates for each subject in test 1 vs test 2 and the standard deviation of 1.1 SPM. Effect size between test 1 and two were also trivial.

Discussion

The optimized peak power protocol has proved to be very reliable as a method of identifying peak power on a cycle, and allowing the accurate identification of the optimal load for each subject to elicit the highest anaerobic power output for cycling, rowing and arm cranking [18,19]. Identification of anaerobic power in swimming has proved to be less than satisfactory previously as identified power through land based exercise (Bio- kinetic swim bench [20], and the MAD system, to name two, has produced values that are significantly below those produced in other sporting types by athletes of a similar level. OPP as a method to assess peak power in swimming had not been attempted previously where identification of anaerobic power has been less reliable.

Time of the swim decayed with the increase in resistance and similarly power recorded increased as the swim time decayed. Although times were significantly different through swim time 1-4 in test 1, there was no significant change in time between swim 3 and 4 in test 2, although power did notably continue to rise significantly where the protocol required a smaller increase in resistance between these swims in both tests. Similarly, stroke rate was significantly different between swims 1 and 3 but not between SR3 and SR4. This was the same in both test 1 and test 2. Power measured with each swim was significantly different between swims 1 through 4 in test 1 and test 2, however in each test a parabola was seen to form in each swimmers data. These results may indicate that time alone is not necessarily an indicator of power production of the swimmer.

Test re-test data showed that there was no significance between test 1 and test 2 in times, stroke rate or power output. Stroke rate ranges were consistent between the two tests. Variation may have occurred through simply learning how to swim the test, but the degree of effect of this appears to be small in the results, and did not have any effect on the outcome. Stroke rate was seen to decrease with the increase in load. Stroke count was seen to increase in some subjects, (stroke length reduction) but not all. There was no significant difference between the calculated OPP in test 1 and test 2 even though we recognize that there was a large difference for some swimmers but within the confidence limits (±77.8 watts at 98% confidence level). The results suggest that there is a good repeatability of the values produced by using the OPP protocol. This is in agreement with studies using sports [21] and across fitness levels also.

In comparison with peak power in other sports, peak power had been investigated by a number of authors [22-25], and have reported power outputs as Watts per kg (W/kg) as being 13.13W/kg (988±39watts) with university students cycling, 11.77 w/kg (849±67 watts) in elite rowing, 10 w/kg in Olympic Speed skaters and 10.4 w/kg in district volley-ballers doing arm cranking. In this study our swimmers mean was test 1 11.0±1 and test2 10.9±1 w/kg (877&864 watts). Our results for swimmers compare favorably to the values found in other sports which is more in line with what might be expected. This is clearly different from what has been found in other studies of power output in swimmers [26,27]. The measurement of force by these authors clearly has not measured power as it is understood in other sports. In the case of Sharp et al. [20], the bio-kinetic swim bench measured upper body force with a fixed body. This may be able to create reliable results, however it does not seem to be relevant to power that was measured in the present study. Similarly, the forces identified by the MAD system also do not seem to relate to the values seen in other sports, or in the present study.

Conclusion

Peak power is known to be an important predictor of swimming performance in 50m and 400m events, although measurement of power in swimming has previously produced varied results that have not been comparable to other sports. The present study evaluated the potential to use the OPP test in the pool situation with elite swimmers using the protocol copied from that of Winter 1995. Consistent results were achieved in test re test values, and the relationship between power measured in our group of elite swimmers and previous tests in other sports appeared to be more in line. We conclude that the OPP test used with swimmers is a far more appropriate measure of power than previous tests used with swimming.