Investigation of Shear Capacities of Cold-Formed Steel Channel & SupaCee Sections
Ngoc Hieu Pham*
Department of Civil Engineering, Hanoi Architectural University, Vietnam
Submission: August 18, 2021; Published: December 06, 2021
*Corresponding Author: Ngoc Hieu Pham, Department of Civil Engineering, Hanoi Architectural University, Vietnam
How to cite this article:Ngoc Hieu Pham, Investigation of Shear Capacities of Cold-Formed Steel Channel & SupaCee Sections.Civil Eng Res J. 2021; 12(4): 555844. DOI 10.19080/CERJ.2021.12.555844
Abstract
Cold-formed steel channel sections have been widely applied in structural buildings. This type of section is then added stiffeners in the web to form the new section called SupaCee. The capacities of these sections have been investigated due to shear actions paralleling their webs. Shear forces are assumed to be resisted by the web, but the presence of the flanges and lips also have specific impacts on the shear capacities of these sections. This paper, therefore, investigates the effects of the flange widths and lip lengths on the shear capacities of the channel and SupaCee sections. Also, the shear capacities of SupaCee sections are studied to illustrate their strength improvements compared to those of channel sections. Shear capacities of the investigated sections are determined according to AS/NZS 4600:2018. The study demonstrated the innovation of SupaCee sections in shear strength improvements in comparison with those of channel sections.
Keywords: Shear capacities; Cold-formed steel; Channel sections; SupaCee sections.
Introduction
Cold-formed steel channel sections have become a common product in structural buildings with numerous applications [1]. They can be subjected to compression, bending or shear. In terms of compression or bending, buckling modes such as local, distortional, flexural, or flexural-torsional buckling have been investigated in many research studies and are deeply understood [1]. In the case of shear, shear buckling of channel section was investigated with the consideration of the web alone, but the flanges and the lips were ignored in the behavior. There was not any consistent theory for shear buckling of full thin-wall sections. The channel sections have then added web stiffeners to increase the stabilities. These such sections termed as SupaCee sections have a variety of advantages compared to the traditional channel ones, as discussed in Pham and Vu [2]. The recent development of the DSM method for thin-wall sections in shear required the elastic buckling loads of the whole section in pure shear. Pham and Hancock [3-6] carried out a series of the channel and SupaCee section beams under shear to provide deep understandings of their strengths and behaviors. Also, Hancock and Pham [7,8] used the complex Semi-Analytical Finite Strip Method proposed by Plank and Wittrick [9] to develop the signature curve for channel sections under shear actions with the assumption of unrestraint at end conditions. Pham and Hancock [10] used a spline finite strip analysis to investigate the shear buckling of whole channel sections restrained at their ends. The spline finite strip analysis was developed by Lau and Hancock [11]. To reduce computer resources in analysis, Hancock and Pham [12] developed a new version of the semi-analytical finite strip analysis called reSAFSM that allows considering restrained ends in the analysis of thin-walled sections under shear actions. Channel sections with intermediate web stiffeners have been also investigated by Pham [13-14] using Semi-Analytical Finite Strip Method. This method was subsequently incorporated into the Thin-Wall-2 [15] in the analysis of the buckling loads under shear [16]. Thin-Wall-2 has been allowed to use for elastic buckling analysis of thin- wall sections according to the AS/NZS 4600-2018 [17].
The paper, therefore, is aimed to investigate the shear capacities of the channel and SupaCee sections with the variations of dimensions of the flanges and lips. The effects of the flanges and lips on the elastic shear buckling loads of thin-walled channel sections can be accounted for as presented in Appendix D3, but the intermediate web stiffeners are not included. Thin-Wall-2 software program [15], therefore, can be used for the elastic shear buckling analyses in this investigation. The shear capacities of commercial SupaCee sections are also investigated to illustrate their innovation in strength improvements based on comparing the shear capacities between SupaCee and channel sections. The investigated sections are commercial sections provided by BlueScope Lysaght [18]. The THIN-WALL-2 software program [15] is used for elastic bucking analysis under shear actions, and the shear capacities are determined according to the AS/NZS 4600: 2018 [17].
Determination of the Shear Capacities of Cold- Formed Steel Sections
The nominal shear capacity of beams without transverse web stiffeners can be calculated determined according to AS/NZS 4600-2018 [17] as follows:
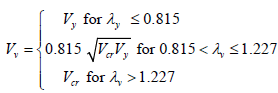
where 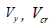 are the yield shear force and the elastic shear
buckling force of the section;
are the yield shear force and the elastic shear
buckling force of the section;
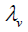 is the non-dimensional slenderness,
is the non-dimensional slenderness, 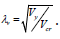
The yield shear force  , can be calculated as
, can be calculated as 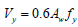 , where
, where  is the area of the web element; y f is the design yield
stress. The elastic shear buckling force
is the area of the web element; y f is the design yield
stress. The elastic shear buckling force 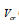 can be determined
according to Appendix D, AS/NZS 4600-2018 [17] or a rational
elastic buckling analysis. THIN-WALL-2 software program [15]
will be used for this elastic buckling analysis in this investigation.
This software program was developed at the University of Sydney
using the finite strip method. One of the outputs of buckling
analysis is a signature curve performing the relationship between
the elastic buckling stress and the half-wavelength of each
buckling mode. This program can be used for buckling analysis
under compression, bending, shear, localized loading or combined
actions. (Figure 1 (a)) shows the signature curve of a channel
section under the shear actions paralleling the web. The elastic
shear buckling stress is the minimum point in the curve with the
buckling mode demonstrated in (Figure 1(b)).
can be determined
according to Appendix D, AS/NZS 4600-2018 [17] or a rational
elastic buckling analysis. THIN-WALL-2 software program [15]
will be used for this elastic buckling analysis in this investigation.
This software program was developed at the University of Sydney
using the finite strip method. One of the outputs of buckling
analysis is a signature curve performing the relationship between
the elastic buckling stress and the half-wavelength of each
buckling mode. This program can be used for buckling analysis
under compression, bending, shear, localized loading or combined
actions. (Figure 1 (a)) shows the signature curve of a channel
section under the shear actions paralleling the web. The elastic
shear buckling stress is the minimum point in the curve with the
buckling mode demonstrated in (Figure 1(b)).

Effects of Flanges and Lips on the Shear Capacities of Channel and SupaCee Sections
The geometry of the unlipped, lipped channel and SupaCee
sections are demonstrated in (Figure 2). The section depths (D)
are 250 mm, the flanges (B) vary from 0.1 to 0.9 times of the depth
(D). The sectional thicknesses (t) are 1.5 mm, 1.9 mm and 2.4 mm,
and the inner radii at corners (r1 or r2) are 5 mm. The elastic shear buckling stresses are determined with the support of the THINWALL-
2 software program [15]. The buckling stresses are listed
in (Table 1) with the variation of the dimensions of the flanges
and the lips. The shear capacities are then calculated with the
design yield stress  of 450 MPa, as presented in Section 2. The
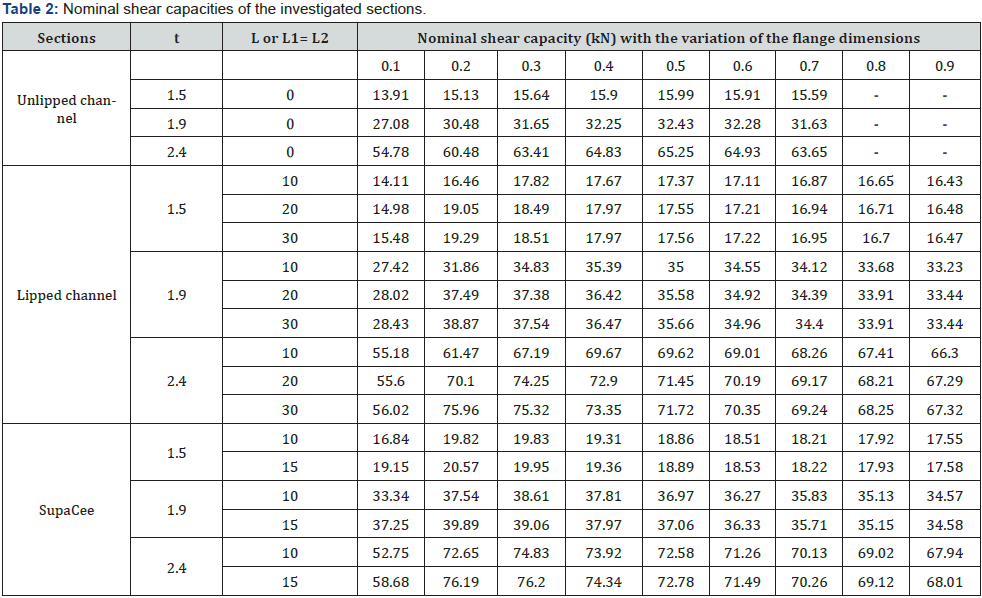
nominal shear capacities are listed in (Table 2) and demonstrated
in (Figure 3).
of 450 MPa, as presented in Section 2. The
nominal shear capacities are listed in (Table 2) and demonstrated
in (Figure 3).




Based on the results in (Table 2), the shear forces are maximum at the ratio B/D of 0.5 for unlipped channel sections, whereas the maximum of shear forces are obtained at the lower ratio B/D varying from 0.2 to 0.4 depending on the lip lengths for lipped channel or SupaCee sections. In terms of lipped channel and SupaCee sections, as the ratio B/D increases from 0.1 to 0.2, the shear force goes up rapidly. This illustrates the significant contribution of the flange on the shear capacities of the channel sections. The ratio B/D subsequently increases, the shear capacities improve slowly to the maximum values, subsequently reducing gradually as the flange width increase to 0.9. These results can be explained due to the effects of flange slenderness (Figures (4&5)). With the small flange, there is very little effect of flange slenderness on the shear buckling capacity. This effect is significant when the flange dimensions increase resulting in the reduction of the shear capacities. In terms of the unlipped channel section, as the increase of the ratio B/D, the shear capacities have a slow increase trend to the maximum values, and then have a gradual reduction. When the ratio B/D increases to 0.8 and 0.9, shear buckling occurs in the flanges (Figure 6), this leads to the significant reductions of elastic buckling stresses. The lips are then added, shear buckling is prevented in the flanges, and the elastic shear buckling stresses are significantly improved as presented in (Table 1).


Comparison of Shear Capacities of Channel and SupaCee Sections
The channel and SupaCee sections for this investigation are
taken from the catalogue provided by BlueScope Lysaght [18].
Their dimensions are presented in (Table 3) with the nomenclature
demonstrated in (Figure 2). The elastic shear buckling stresses
are determined using the Thin-Wall-2 software program [15] and
are listed in (Table 3). These buckling stresses are then used to determine shear capacities of the investigated sections according
to AS/NZS 4600-2018 [17] as presented in Section 2. The design
yield stress 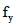 of 450 MPa is used for this investigation. Shear
capacities of channel and SupaCee are summarized in (Table 3).
The results of shear capacities are plotted in percentage diagrams,
where the shear capacities of channel sections are shown in the
horizontal axis and the vertical axis is for the shear capacity
deviations (in %) between SupaCee and channel sections, as
illustrated in (Figure 7).
of 450 MPa is used for this investigation. Shear
capacities of channel and SupaCee are summarized in (Table 3).
The results of shear capacities are plotted in percentage diagrams,
where the shear capacities of channel sections are shown in the
horizontal axis and the vertical axis is for the shear capacity
deviations (in %) between SupaCee and channel sections, as
illustrated in (Figure 7).

The SupaCee sections have demonstrated their innovation in shear capacities compared to those of channel sections due to the effects of the intermediate web stiffeners with the increase of shear capacities reaching 22%. The web stiffeners become more beneficial effects with the smaller thicknesses in all investigated sections. This conclusion can be seen as the strength improvements of the SC250 section decrease from 11.54% to 4.48% when the thicknesses increase from 1.5mm to 2.4mm as presented in (Table 4). This trend is also observed in the other sections. For small sections (SC150 and SC200 sections), the shear strength improvements are more noticeable for the small section. This result is illustrated as the higher strength improvements of SC150 section compared to those of SC200 section in almost investigated thicknesses. For large sections (see SC250 to SC400 sections), the strength improvements become more significant as the increase of web slenderness. This conclusion is based on the increasing trend of strength improvements from 7.29% to 9.14% corresponding to the increase of web slenderness from SC25019 to SC40019 sections. This trend is also seen for the other thicknesses.

Note: the inner radius r1 = r2 = 5mm; t, D, B, L1, L2, GS, S (mm); α1, α2(0)
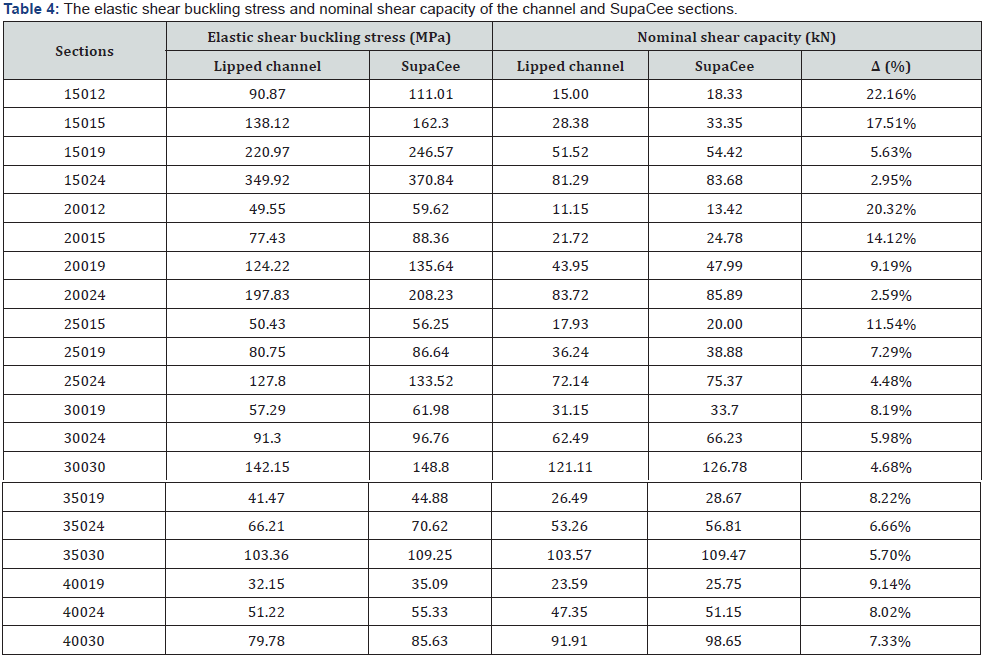
Note: Δ (%) is the nominal shear capacity deviation between SupaCee and Lipped channel sections (in %)
Conclusion
The paper investigated the effects of flange widths and lip lengths on the shear capacities of unlipped channel, lipped channel and SupaCee sections. The investigated results have demonstrated the role of the flanges and lips on the shear capacities with the significant increase of shear forces as the ratio of B/D increase from 0.1 to 0.2, but then observe a gradual reduction due to the effects of flange slenderness. Also, the lips can prevent the shear buckling from occurring in the flanges with the large ratio of B/D. The paper subsequently investigated the innovation in shear capacities of SupaCee sections compared to those of traditional channel sections with significant shear strength improvements.
References
- Yu WW, Laboube RA, Chen H (2019) Cold-formed Steel Design. John Wiley and Sons, 111 River Street, Hoboken, NJ 07030, USA.
- Pham NH, Vu QA (2021) Effects of stiffeners on the capacities of cold-formed steel channel members. Steel Construction 14(4): 270-278.
- Pham CH, Hancock GJ (2010) Direct strength design of cold-formed C-sections in combined bending and shear. 20th International Specialty Conference on Cold-Formed Steel Structures-Recent Research and Developments in Cold- Formed Steel Design and Construction pp: 221-236.
- Pham CH, Hancock GJ (2010) Finite element analyses of high strength Cold- Formed SupaCee® Sections in Shear, Proceedings of SDSS Rio. International Colloquium Stability and Ductility of Steel Structures Volume 2, 1025-1032.
- Pham CH, Hancock GJ (2013) Experimental Investigation and Direct Strength Design of High-Strength, Complex C-Sections in Pure Bending, Journal of Structural Engineering 139(11): 1842-1852.
- Pham CH and Hancock GJ (2012) Direct strength design of cold-formed sections for shear and combined actions. Journal of Structural Engineering Volume 1, 759-768.
- Hancock GJ, Pham CH (2011) A signature curve for cold-formed channel sections in pure shear. Research Report R919.
- Hancock GJ, Pham CH (2012) Direct method of design for shear of cold-formed channel sections based on a shear signature curve. in 21st international specialty conference on cold-formed steel structures pp: 207-221.
- Plank RJ, Wittrick WH (1974) Buckling under Combined Loading of Thin, Flat- Walled Structures by a Complex Finite Strip Method. International Journal for Numerical Methods in Engineering 8(2): 323-339.
- Pham CH, Hancook GJ (2009) Shear buckling of thin-walled channel sections. Journal of Constructional Steel Research. 65(3): 578-585.
- Lau SCW, Hancock GJ (1986) Buckling of thin-flat-walled structures by a spline finite strip method. Thin-Walled Structures 4(4): 269-294.
- Hancock GJ, Pham CH (2013) Shear buckling of channel sections with simply supported ends using the Semi-Analytical Finite Strip Method. Thin-Walled Structures 71: 72-80.
- Pham SH, Pham CH, Hancock GJ (2012) Shear buckling of thin-walled channel sections with complex stiffened webs. Research Report R924.
- Pham SH, Pham CH, Hancook GJ (2012) Shear buckling of thin-walled channel sections with complex stiffened webs. in 21st international specialty conference on cold-formed steel structures pp: 281-296.
- Nguyen VV, Hancock GJ, Pham CH (2015) Development of the Thin-Wall- 2 for Buckling Analysis of Thin-Walled Sections Under Generalized Loading. in Proceeding of 8th International Conference on Advances in Steel Structures.
- Hancock GJ, Pham CH (2022) Finite strip methods for stability analysis of thin- walled members with applications to the Direct Strength Method of design LTD.
- (2018) AS/NZS 4600-2018, Australian / New Zealand Standard TM Cold-formed steel structures. The Council of Standards Australia.
- (2014) BlueScope Lysaght, Supapurlins Supazeds & Supacees. Blue Scope Lysaghts.






























